In this fascinating paper, Caleb Scharf and Olaf Witkowski make use of the fact that one main biological process is to carry out computation to establish new bounds on the traditional habitable zone, which they term the computational zone.

They also discuss at length and more generally the constraints on computation in physical systems as a means to derive theoretical limits on suitable environments for living systems.

The paper opens on a discussion of the traditional concept of the habitable zone, defined as the temperature on an exoplanet where water can be in its liquid state. The authors highlight some of its shortcomings, such as the fact that estimating the bounds of the habitable zone sometimes depends on factors that are poorly constrained, which allows them to introduce their main idea: given the fact that computational abilities could be one of the hallmarks of living systems, we can use this information in life detection missions to conceptualize a new type of habitable zone, which would be more general in scope than the traditional habitable zone that assumes life’s requirement of liquid water.

In other words, one of the advantages of basing our search for life on those computational abilities of living systems is that it is thought to be agnostic; this ability for life to carry out computation would be the same whether life is instantiated as carbon-based or any other type of system, and could even extend to any type of technological evolution.

Defining computation in a general way, as the “systematic manipulation of information, following a well-defined set of rules”—a definition owed to Stephen Wolfram—we can thus generalize the concept of habitable zone and define “computational zones” in a similar manner as the environments that could support computation in living systems. This computational zone would likely to be modulated as a function of available energy, energy utilization efficiency and many other factors.

As a prelude to this investigation of the concept of computational zone, Scharf and Witkowski begin by analyzing what factors can act as constraints to biological computation. They identify three types of constraints:

These constraints would likely be universal and thought to apply to any physical system carrying out any type of computation. Now in what way can these help us define the computational zone? For simplicity, the authors thereafter focus on three main limiting factors:

On the basis of these factors, we can now try to derive some general relations that could underly a new concept of habitable zone.

So basically what we will want to do here is to try to derive a set of limiting boundaries—or optimal parameters—for computation to take place. Assuming that the Landauer limit we just mentioned is an absolute one, Scharf and Witkowski suggest that for a process labelled \(x\) the energy requirement for computation to occur should be \(a_xE_\text{landauer}\) where \(a_x\) would depend on the specific biological process at play.

This allows them to define a new quantity, the maximum number of biological operations per second for process \(x\), termed \(BOPS_x\) as:

$$ B O P S_x \leq \frac{1}{\alpha_x E_{\text {landauer }}} \varepsilon_x P \qquad \qquad (1) $$

with \(P\) being the power flow in the system and \(\varepsilon_x\) a thermodynamic efficiency factor representing the available energy. Skipping some of the physics here, they also derive an equation for the efficiency factor \(\varepsilon\):

$$ \varepsilon=1-\frac{4 T_0}{3 T}+\frac{1}{3}\left(\frac{T_0}{T}\right)^4 \qquad \qquad (2) $$

We can see that there is a dependency on the environmental temperature \(T_0\), which could be the temperature on a given planet where life could eventually be sustained, and the blackbody radiation \(T\), which in this context would be the temperature of the host star. Those are the two main equations that will be used to derive new bounds on their computational zone.

We just saw that the equations in the previous section depended on several parameters. Fortunately, these parameters can be derived from known astrophysical relations. For example, the temperature \(T_0\) of a planet and thermodynamic efficiency \(\varepsilon\) can be cast in terms of the planet-star separation \(d\), which is the parameter we’re looking to constrain with the concept of habitable (or computational) zone. Using these relationships allows us to finally derive the maximum number of biological operations per second, \(BOPS\), for any given planet-star separation. For a more realistic account, Scharf and Witkowski introduce a temperature dependent efficiency factor, since we know that terrestrial biochemical processes are dependent on the temperature. Our new computational zone is plotted on the next figure, with the dashed red curve indicating a cool (M dwarf) star while the blue curve represents a solar-type star.

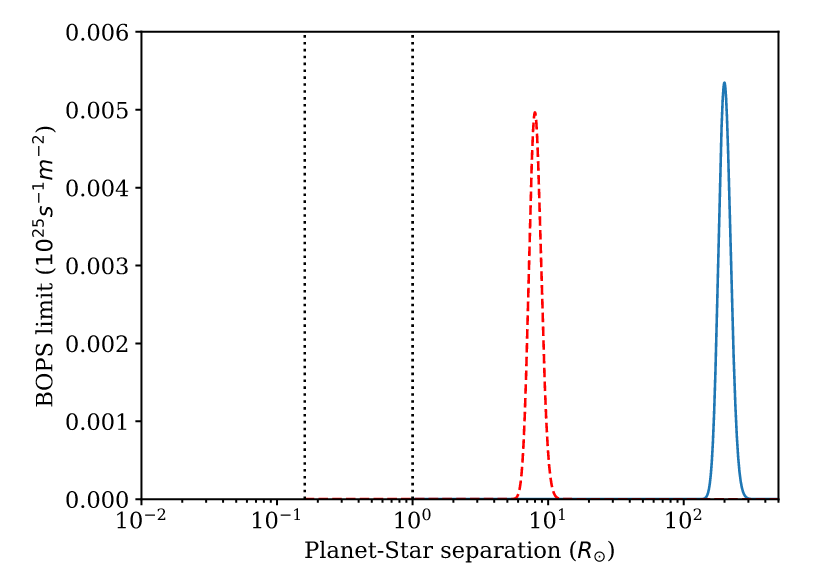
We can see that for the solar-type star, this computational zone would likely be around 200 solar radii, which is pretty close to the actual separation between the Earth and the Sun at around 215 solar radii. So at first glance, the computational zone agrees with the habitable zone for our own solar system, which is obviously a good thing.

Derived in this way, the computational zone can thus be seen as a generalization of the traditional habitable zone. While the latter depends on a particular biochemistry (ours), the computational zone is assumed to only depend on a universal feature of life that isn’t correlated in any way with a particular biochemistry. The authors note the similarity between this type of approach and that of assembly theory (see e.g. the work of Marshall (2021)), which is based on conceptualizations of life that strive to be universal.

As closing remarks, Scharf and Witkowsi mention—speculatively—that advanced technological species might realize how limiting biological computation is to the availability of suitable environments, and as such may transition towards other “computational modes of existance”. Imagining what those are is left as an exercise to the reader!